
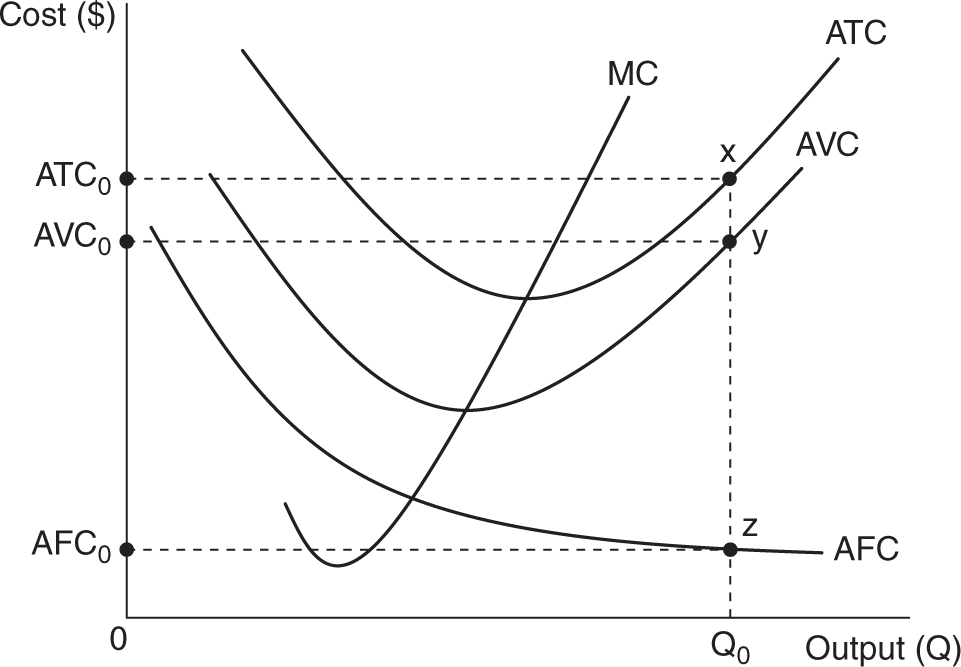
In empirical work it is sometimes easier to estimate a revenue function than a demand function. This MC = MR method is often useful in the analysis of firms’ behaviour. The other form can be illustrated by drawing the demand and isoprofit curves, and showing the tangency point. MC = MR is illustrated by drawing the MC and MR curves and finding the intersection. We drew very different diagrams to illustrate the two forms of the first-order condition. Remember that it can be interpreted as saying that the slope of the demand curve is equal to the slope of the isoprofit curve. Which is the first-order condition of Leibniz 7.5.1. Thus the first-order condition may be written: Using the rule for differentiating a product to differentiate, we see that: We now show that the first-order condition for profit maximization derived above,, is equivalent to the first-order condition for profit maximization given in Leibniz 7.5.1. In general, if we can find a solution to the first-order condition MC = MR, we can say that it is the profit-maximizing quantity if MC MR when. If MC sloped downward (which can happen, if the firm has economies of scale) and MR sloped upward (which would be unusual, but can happen for some demand functions), the point of intersection would be a profit- minimizing point (try drawing the curves and explaining to yourself why this must be true). As explained in the interactive for Figure 7.12b in the text, we can see that this is profit-maximizing by noting that the marginal cost of producing more than 32 cars would be greater than the marginal revenue generated (MC > MR), while the opposite would be true if fewer than 32 were produced.īut note that if the curves had sloped differently this argument might not have worked. Since Beautiful Cars has an upward-sloping MC and downward-sloping MR, there is just one point of intersection.Īt point E, the company produces 32 cars. The profit-maximizing quantity lies at the point where the two curves cross-at point E in Figure 2, where. Figure 1 reproduces the middle panel of Figure 7.12b from the text, showing both curves. In the text we drew the MR curve as downward-sloping. Similarly, the function is the marginal revenue curve, showing how marginal revenue changes with output. In the case of Beautiful Cars, we know that marginal cost increases with output, so the MC curve is upward-sloping. The marginal cost curve (that is, the function ) shows how marginal cost changes as output changes. So the first-order condition tells us that, when is at its profit-maximizing level, the marginal revenue is equal to the marginal cost. Thus, the first-order condition for profit maximization may be written as: Similarly, the derivative of the revenue function, is the rate at which revenue rises with output, and it is known as marginal revenue (MR). The term on the right-hand side of the equation is the firm’s marginal cost (MC)-the rate at which cost increases as output rises. marginal revenue The change in revenue obtained by increasing the quantity from Q to Q + 1. It corresponds to the slope of the total cost function at each point. To find the value of that maximizes profit, we differentiate with respect to, to obtain the first-order condition, which implies that: marginal cost The effect on total cost of producing one additional unit of output. Buy with confidence.The expression for profits, above, can be written as a function of output, as the difference between the total revenue function and total cost : These ATCs are highly analytical yet never sound clinical or passionless, and are transparent to the original recording and system electronics. Prince’s vocals are handled in a crisp and articulate manner, but they also lack nothing in terms of body or natural warmth.
The bass kicks hard while the drum machines attack with determination. They deliver the song’s relentless rhythmic momentum with enthusiasm.

Moving onto Prince’s Love shows that these boxes like to party too.

Things stay stable even as the piece’s complexity increases. We also like the SCM19s’ ability to render a large-scale soundstage and populate it with well-focused instruments. There’s an impressive degree of composure here, the monitors refusing to compress or harden up even when pushed hard.
These ATCs have no trouble delivering the piece’s crescendos with power, even at very high volume levels. Those dynamic skills come into even sharper focus when we play Tchaikovsky’s 1812 Overture. Twin binding posts allow biamping or biwiring if required.


 0 kommentar(er)
0 kommentar(er)
